Frequencies
A frequency is the speed at which a sound wave vibrates. This wave speed is measured through the number of oscillations or cycles per second that a wave has and its unit of measurement is the Hertz (Hz).
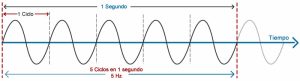
Frequency determines the pitch or note of the sound we perceive: the higher the frequency, the faster the oscillation of the wave, the higher the pitch of the sound; the lower the frequency, the slower the oscillation of the wave, the lower the pitch of the sound.

As we can see in the following graph, sound A (0r4) is lower than sound B (9r4), since it has a lower vibration frequency.
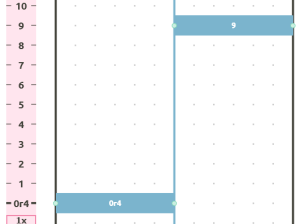
| sound A:
N (0r4) = 261 Hz   |
sound B:
N (9r4) = 440 Hz   |
To produce a sound, whether it is a musical note or any other type of sound that can be integrated into our compositions, we have to apply force to some object so that it emits a vibration or sound wave. When we vibrate any object, be it the string of a musical instrument, a table that we have given a small blow, or any everyday physical object, not only do we physically vibrate the object on which we have applied the force, but we also make the air molecules around it vibrate, creating sound waves that propagate through the air until they reach our ears.
Vibrations can also be produced through electronics, as would be the case of the oscillator of an analog synthesizer or the transistor of an amplifier or any speaker that transforms an electrical signal into a vibration that reaches our ears as sound.
Therefore, sounds are vibrations that we measure in frequencies and to which we assign musical notes. Each note is linked to a certain frequency, for example:
Note A= 440 Hz N (9r4)
A musical note is a very special type of sound, the result of very specific characteristics. We can define two types of note: the simple note and the complex note. The simple note is the result of a single wave and is virtually impossible to find in a natural environment. The complex note is the result of the interaction between several simple waves and it is precisely its complexity that gives it its sonorous richness, or timbre.
 |
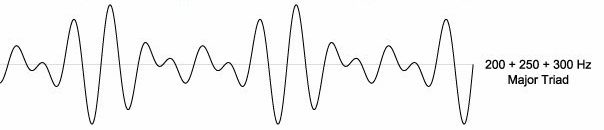 |
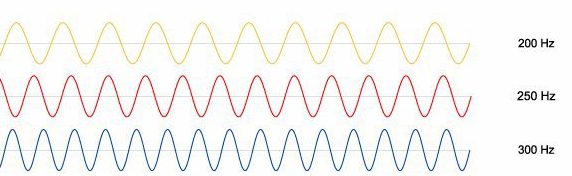
In the graph on the left we can see three of the simple waves that form a sound, each in a different color. In the graph on the right we can see the shape of the complex wave resulting from the interaction between the three simple waves.
When we hear a note produced by some kind of acoustic instrument, several frequencies are actually sounding at the same time, although we do not necessarily perceive it that way. The frequency we hear most clearly is the fundamental note. This note is the result of the vibration that has the greatest amplitude, that is, the most audible, and the one that gives it its name (C, D, E…). The rest of the notes that form the complex note, called the harmonic series, are the result of other simple waves produced by secondary vibrations of the same instrument (as for example in the string of a guitar).. These secondary waves or harmonics are almost inaudible as they have a very low amplitude.
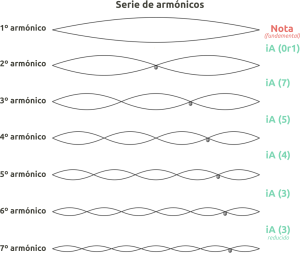
Depending on the harmonics that have greater amplitude, we generate one type of timbre or another. This is what explains that although a violin and a piano play exactly the same note, we perceive a different sound or timbre.